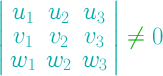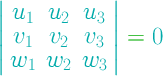Dependencia e independencia lineal
De Wikillerato
m (Revertidas las ediciones realizadas por 190.26.230.40 (Talk); a la última edición de 201.204.3.130) |
|||
| (19 ediciones intermedias no se muestran.) | |||
| Línea 1: | Línea 1: | ||
| + | ==Combinación lineal== | ||
| + | |||
| + | <br/> | ||
| + | |||
Una combinación lineal de los vectores | Una combinación lineal de los vectores | ||
<math> | <math> | ||
| Línea 9: | Línea 13: | ||
<center> | <center> | ||
<math> | <math> | ||
| - | \alpha_1 \cdot \vec{\mathbf{v}}_1 \, + \, \alpha_2 \cdot \vec{\mathbf{v}}_2 \, + \, \ldots \, + \, | + | \alpha_1 \cdot \vec{\mathbf{v}}_1 \, + \, \alpha_2 \cdot \vec{\mathbf{v}}_2 \, + \, \ldots \, + \, \alpha_n \cdot \vec{\mathbf{v}}_n |
| - | \alpha_n \cdot \vec{\mathbf{v}}_n | + | |
</math> | </math> | ||
</center> | </center> | ||
| Línea 24: | Línea 27: | ||
<br/> | <br/> | ||
| - | + | ===Ejemplo=== | |
<br/> | <br/> | ||
| Línea 43: | Línea 46: | ||
<br/> | <br/> | ||
| - | + | ==Independencia lineal== | |
<br/> | <br/> | ||
| Línea 57: | Línea 60: | ||
<center> | <center> | ||
<math> | <math> | ||
| - | \alpha_1 \vec{\mathbf{v}}_1 \, + \, \alpha_2 \vec{\mathbf{v}}_2 \, + | + | \alpha_1 \vec{\mathbf{v}}_1 \, + \, \alpha_2 \vec{\mathbf{v}}_2 \, + \, \ldots \, + \, |
\alpha_n \vec{\mathbf{v}}_n \, = \, 0 \, \Rightarrow \, \alpha_1 \, = \, \alpha_2 \, = \, | \alpha_n \vec{\mathbf{v}}_n \, = \, 0 \, \Rightarrow \, \alpha_1 \, = \, \alpha_2 \, = \, | ||
\ldots \, = \, \alpha_n \, = \, \ldots \, = \, 0 | \ldots \, = \, \alpha_n \, = \, \ldots \, = \, 0 | ||
| Línea 65: | Línea 68: | ||
<br/> | <br/> | ||
| - | + | ===Ejemplo=== | |
<br/> | <br/> | ||
| Línea 94: | Línea 97: | ||
<center> | <center> | ||
<math> | <math> | ||
| - | \alpha \vec{\mathbf{u}} \, + \, \beta \vec{\mathbf{v}} \, + | + | \alpha \vec{\mathbf{u}} \, + \, \beta \vec{\mathbf{v}} \, + \, \ldots \, + \, |
\gamma \vec{\mathbf{w}} \, = \, 0 \, \Rightarrow | \gamma \vec{\mathbf{w}} \, = \, 0 \, \Rightarrow | ||
</math> | </math> | ||
| Línea 137: | Línea 140: | ||
\vec{\mathbf{v}}_1, \, \vec{\mathbf{v}}_2, \, \ldots, \, \vec{\mathbf{v}}_n | \vec{\mathbf{v}}_1, \, \vec{\mathbf{v}}_2, \, \ldots, \, \vec{\mathbf{v}}_n | ||
</math> | </math> | ||
| - | son linealmente | + | son linealmente dependientes si existen numeros reales |
<math> | <math> | ||
\alpha_1, \, \alpha_2, \, \ldots \, , \, \alpha_n | \alpha_1, \, \alpha_2, \, \ldots \, , \, \alpha_n | ||
| Línea 147: | Línea 150: | ||
<center> | <center> | ||
<math> | <math> | ||
| - | \alpha_1 \vec{\mathbf{v}}_1 \, + \, \alpha_2 \vec{\mathbf{v}}_2 \, + \, | + | \alpha_1 \vec{\mathbf{v}}_1 \, + \, \alpha_2 \vec{\mathbf{v}}_2 \, + \, \ldots \, + \, |
\alpha_n \vec{\mathbf{v}}_n \, = \, 0 | \alpha_n \vec{\mathbf{v}}_n \, = \, 0 | ||
</math> | </math> | ||
| Línea 154: | Línea 157: | ||
<br/> | <br/> | ||
| - | + | ===Ejemplo=== | |
<br/> | <br/> | ||
| Línea 183: | Línea 186: | ||
<center> | <center> | ||
<math> | <math> | ||
| - | \alpha \vec{\mathbf{u}} \, + \, \beta \vec{\mathbf{v}} \, + | + | \alpha \vec{\mathbf{u}} \, + \, \beta \vec{\mathbf{v}} \, + \, \ldots \, + \, |
\gamma \vec{\mathbf{w}} \, = \, 0 \, \Rightarrow | \gamma \vec{\mathbf{w}} \, = \, 0 \, \Rightarrow | ||
</math> | </math> | ||
| Línea 191: | Línea 194: | ||
<math> | <math> | ||
\left( | \left( | ||
| - | \, \alpha\, - \,\beta \, + \, \gamma, \, \beta \, + \, \gamma, \, \alpha \, + \, 2\gamma \, | + | \, \alpha \, - \, \beta \, + \, \gamma, \, \beta \, + \, \gamma, \, \alpha \, + \, 2\gamma \, |
\right) | \right) | ||
\, = \, | \, = \, | ||
| Línea 197: | Línea 200: | ||
\, 0, \, 0, \, 0 \, | \, 0, \, 0, \, 0 \, | ||
\right) | \right) | ||
| - | |||
| - | |||
</math> | </math> | ||
</center> | </center> | ||
| Línea 263: | Línea 264: | ||
, dos vectores | , dos vectores | ||
<math> | <math> | ||
| - | u \, = \, | + | \vec{\mathbf{u}} \, = \, |
\left( | \left( | ||
\, u_1, \, u_2 \, | \, u_1, \, u_2 \, | ||
| Línea 270: | Línea 271: | ||
y | y | ||
<math> | <math> | ||
| - | v \, = \, | + | \vec{\mathbf{v}} \, = \, |
\left( | \left( | ||
\, v_1, \, v_2 \, | \, v_1, \, v_2 \, | ||
| Línea 283: | Línea 284: | ||
border = "n" > | border = "n" > | ||
<tr> | <tr> | ||
| - | < | + | <td> |
linealmente <span style= 'color:#00AA00'> independientes | linealmente <span style= 'color:#00AA00'> independientes | ||
</span> si: | </span> si: | ||
| - | </ | + | </td> |
| - | < | + | <td> |
linealmente <span style= 'color:#00AA00'> dependientes | linealmente <span style= 'color:#00AA00'> dependientes | ||
</span> si: | </span> si: | ||
| - | </ | + | </td> |
</tr> | </tr> | ||
<tr> | <tr> | ||
| Línea 315: | Línea 316: | ||
, tres vectores | , tres vectores | ||
<math> | <math> | ||
| - | u \, = \, | + | \vec{\mathbf{u}} \, = \, |
\left( | \left( | ||
\, u_1, \, u_2, \, u_3 \, | \, u_1, \, u_2, \, u_3 \, | ||
| Línea 322: | Línea 323: | ||
, | , | ||
<math> | <math> | ||
| - | v \, = \, | + | \vec{\mathbf{v}} \, = \, |
\left( | \left( | ||
\, v_1, \, v_2, \, v_3 \, | \, v_1, \, v_2, \, v_3 \, | ||
| Línea 329: | Línea 330: | ||
y | y | ||
<math> | <math> | ||
| - | + | \vec{\mathbf{w}} \, = \, | |
\left( | \left( | ||
\, w_1, \, w_2, \, w_3 \, | \, w_1, \, w_2, \, w_3 \, | ||
| Línea 341: | Línea 342: | ||
border = "n" > | border = "n" > | ||
<tr> | <tr> | ||
| - | < | + | <td> |
| - | linealmente <span style= 'color:#00AA00'> independientes </span> si: | + | linealmente <span style= 'color:#00AA00'> independientes </span> si: |
| - | </ | + | </td> |
| - | < | + | <td> |
| - | linealmente <span style= 'color:#00AA00'> dependientes </span> si: | + | linealmente <span style= 'color:#00AA00'> dependientes </span> si: |
| - | </ | + | </td> |
</tr> | </tr> | ||
<tr> | <tr> | ||
| Línea 362: | Línea 363: | ||
</table> | </table> | ||
</center> | </center> | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | [[Categoría:Matemáticas]] | ||
Revisión actual
Tabla de contenidos |
Combinación lineal
Una combinación lineal de los vectores
 , es una suma de la forma:
, es una suma de la forma:
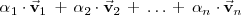
siendo los coeficientes
 numeros reales.
numeros reales.
Ejemplo
Dados los vectores
 y
y
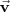 , una combinación lineal de ellos es el vector
, una combinación lineal de ellos es el vector
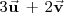
Independencia lineal
Los vectores
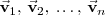 son linealmente independientes si:
son linealmente independientes si:
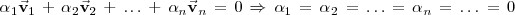
Ejemplo
Los vectores
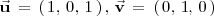 y
y
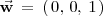 son linealmente independientes, pues:
son linealmente independientes, pues:

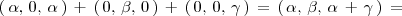
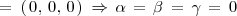
Los vectores
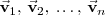 son linealmente dependientes si existen numeros reales
son linealmente dependientes si existen numeros reales
 no todos nulos tales que:
no todos nulos tales que:
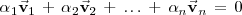
Ejemplo
Los vectores
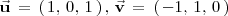 y
y
 son linealmente dependientes, pues:
son linealmente dependientes, pues:

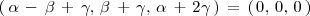
Igualando componentes:
![\left.
</p>
<pre> \begin{array}[c]{rcl}
\alpha\, - \, \beta \, + \, \gamma & = & 0
\\
\beta \, + \, \gamma & = & 0
\\
\alpha \, + \, 2\gamma & = & 0
\end{array}
</pre>
<p>\right\}
</p>
<pre>\, \Rightarrow \beta \, = \, -\gamma, \, \alpha \, = \, -2\gamma
</pre>
<p>
\left.
</p>
<pre> \begin{array}[c]{rcl}
\alpha\, - \, \beta \, + \, \gamma & = & 0
\\
\beta \, + \, \gamma & = & 0
\\
\alpha \, + \, 2\gamma & = & 0
\end{array}
</pre>
<p>\right\}
</p>
<pre>\, \Rightarrow \beta \, = \, -\gamma, \, \alpha \, = \, -2\gamma
</pre>
<p>](/images/math/math-5df83e3ec529fee5da2501449dc541a7.png)
Para cualquier valor que tome
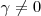 se obtiene un valor para
se obtiene un valor para
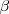 y otro para
y otro para
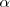 tambien distintos de cero, luego
tambien distintos de cero, luego
 ,
,
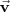 y
y
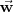 son linealmente dependientes.
son linealmente dependientes.
En
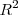 , dos vectores
, dos vectores
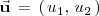 y
y
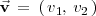 son:
son:
|
linealmente independientes si: |
linealmente dependientes si: |
|
|
|
En
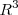 , tres vectores
, tres vectores
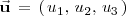 ,
,
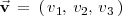 y
y
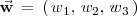 son:
son:
|
linealmente independientes si: |
linealmente dependientes si: |
|
|
|