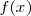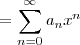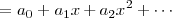Ayuda:Fórmulas Matemáticas

De Wikillerato

Ayuda A continuación ofrecemos un cuadro de referencia con nociones básicas y ejemplos que sirven de ayuda para escribir fórmulas utilizando el código LaTeX.

Básicos

Acentos
\acute{a} \grave{a} \hat{a} \tilde{a} \breve{a}
\check{a} \bar{a} \ddot{a} \dot{a}
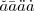Funciones estándar
\sin a \cos b \tan c
\sec d \csc e \cot f
\arcsin h \arccos i \arctan j
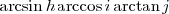\sinh k \cosh l \tanh m \coth n
\lim u \limsup v \liminf w \min x \max y
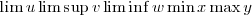\inf z \sup a \exp b \ln c \lg d \log e \log_{10} f \ker g
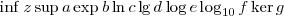Derivadas
\nabla \partial x dx \dot x \ddot y
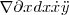Conjuntos
\forall \exists \emptyset \varnothing
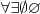\in \ni \notin \subset \subseteq \supset \supseteq
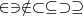\cap \bigcap \cup \bigcup \biguplus \setminus \smallsetminus
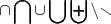\sqsubset \sqsubseteq \sqsupset \sqsupseteq \sqcap \sqcup \bigsqcup
Operadores
+ \oplus \bigoplus \pm \mp -
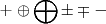\times \otimes \bigotimes \cdot \circ \bullet \bigodot
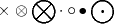\star * / \div \frac{1}{2}
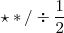Lógica
\land \wedge \bigwedge \bar{q} \to p
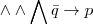\lor \vee \bigvee \lnot \neg q \And
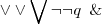Raíces
\sqrt{2} \sqrt[n]{x}
Relaciones
\sim \approx \simeq \cong
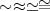\le < \ll \gg \ge > \equiv \not\equiv \ne \mbox{or} \neq \propto
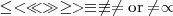Geometría
\Diamond \Box \triangle \angle \perp \mid \nmid \| 45^\circ
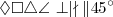Flechas
\leftarrow \gets \rightarrow \to \not\to \leftrightarrow \longleftarrow \longrightarrow
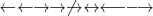\uparrow \downarrow \updownarrow \rightharpoonup \rightharpoondown \leftharpoonup \leftharpoondown \upharpoonleft
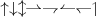\upharpoonright \downharpoonleft \downharpoonright \rightleftharpoons \Leftarrow \Rightarrow \Leftrightarrow \Longleftarrow
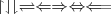\Longrightarrow \Uparrow \Downarrow \Updownarrow
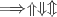\nLeftrightarrow \longleftrightarrow
Especial
\eth \S \P \% \dagger \ddagger \ldots \cdots
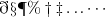\smile \frown \wr \triangleleft \triangleright \infty \bot \top
\vdash \vDash \Vdash \models \lVert \rVert \imath \hbar
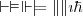Otros
\vartriangle \triangledown \lozenge \circledS \measuredangle \nexists \Bbbk \backprime \blacktriangle \blacktriangledown
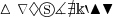\blacksquare \blacklozenge \bigstar \sphericalangle \diagup \diagdown \dotplus \Cap \Cup \barwedge
\veebar \doublebarwedge \boxminus \boxtimes \boxdot \boxplus \divideontimes \ltimes \rtimes \leftthreetimes
\rightthreetimes \curlywedge \curlyvee \circleddash \circledast \circledcirc \centerdot \intercal \leqq \leqslant
\eqslantless \lessapprox \approxeq \lessdot \lll \lessgtr \lesseqgtr \lesseqqgtr \doteqdot
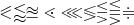\fallingdotseq \backsim \backsimeq \subseteqq \Subset \preccurlyeq \curlyeqprec \precsim \precapprox
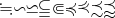\Vvdash \bumpeq \Bumpeq \geqq \geqslant \eqslantgtr \gtrsim \gtrapprox \eqsim \gtrdot
\ggg \gtrless \gtreqless \gtreqqless \eqcirc \circeq \triangleq \thicksim \thickapprox \supseteqq
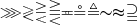\Supset \succcurlyeq \curlyeqsucc \succsim \succapprox \vartriangleright \shortmid \shortparallel \between \pitchfork
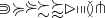\varpropto \blacktriangleleft \therefore \backepsilon \blacktriangleright \because \nleqslant \nleqq \lneq \lneqq
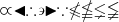\lvertneqq \lnsim \lnapprox \nprec \npreceq \precneqq \precnsim \precnapprox \nsim \nshortmid
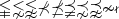\nvdash \nVdash \ntriangleleft \ntrianglelefteq \nsubseteq \nsubseteqq \ngtr
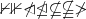\ngeqslant \ngeqq \gneq \gneqq \gvertneqq \gnsim \gnapprox \nsucc \nsucceq \succneqq
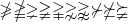\succnsim \succnapprox \ncong \nshortparallel \nparallel \nvDash \nVDash \ntriangleright \ntrianglerighteq \nsupseteq
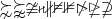\nsupseteqq \varsupsetneq \supsetneqq \varsupsetneqq

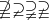Subíndices, superíndices, integrales

	Sintaxis	Cómo se verá
Superíndice	a^2
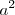Subíndice	a_2
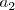Agrupar	a^{2+2}
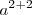	a_{i,j}
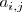Combinar superindice y subíndice	x_2^3
Superíndices y subíndices, anteriores, posteriores, arriba y abajo	\sideset {_1^2} {_3^4} \prod_a^b
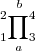	{}_1^2 \! \Omega_3^4
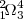Apilar	\overset { \alpha} { \omega}
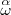	\overset { \alpha} { \underset { \gamma} { \omega}}
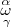	\stackrel { \alpha} { \omega}
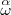Derivadas	x', y, f', f
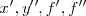Subrayado, línea superior, vectores	\hat a \ \bar b \ \vec c
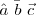	\overrightarrow {a b} \overleftarrow {c d} \widehat {d e f}
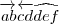	\overline {g h i} \underline {j k l}
Flechas	A \xleftarrow {n+ \mu-1} B \xrightarrow[T] {n \pm i-1} C
Llaves superiores	\overbrace{ 1+2+ \cdots+100 } ^ {5050}
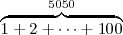Llaves inferiores	\underbrace { a+b+ \cdots+z }_{26}
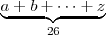Sumatorios	\sum_{k=1}^N k^2
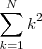Productorio	\prod_{i=1}^N x_i
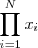Coproducto	\coprod_{i=1}^N x_i
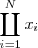Límite	\lim_{n \to \infty}x_n
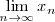Integral	\int_{-N}^{N} e^x\, dx
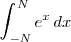Integral doble	\iint_{D}^{W} \, dx\,dy
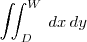Integral triple	\iiint_{E}^{V} \, dx\,dy\,dz
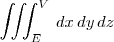Integral de línea	\oint_{C} x^3\, dx + 4y^2\, dy
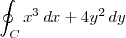Intersecciones	\bigcap_1^{n} p
Uniones	\bigcup_1^{k} p

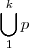Fracciones, matrices, multilíneas

	Sintaxis	Cómo se verá
Fracciones	\frac{2}{4}=0.5
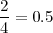Coeficiente binomial	\binom{n}{k}
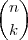Matrices	\begin{matrix} x & y \\ z & v \end{matrix}
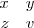	\begin{vmatrix} x & y \\ z & v \end{vmatrix}
	\begin{Vmatrix x & y \\ z & v \end{Vmatrix}
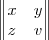	\begin{bmatrix} 0 & \cdots & 0 \\ \vdots & \ddots & \vdots \\ 0 & \cdots & 0 \end{bmatrix}
	\begin{Bmatrix} x & y \\ z & v \end{Bmatrix}
	\begin{pmatrix} x & y \\ z & v \end{pmatrix}
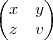	\bigl( \begin{smallmatrix} a&b\\ c&d \end{smallmatrix} \bigr)
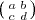Distinción de casos	f(n) = \begin{cases} n/2, & \mbox{if }n\mbox{ is even} \\ 3n+1, & \mbox{if }n\mbox{ is odd} \end{cases}
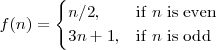Ecuaciones multilínea (se debe definir el número de columnas con {lcl})	\begin{array}{lcl} z & = & a \\ f(x,y,z) & = & x + y + z \end{array}
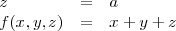	\begin{array}{lclcl} z & = & a & = & \sqrt 2\\ f(x,y,z) & = & x + y + z & = & t^2\\ f(z) & = & x+y & = & 2 \pi \end{array}
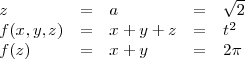Romper largas expresiones para hacer más legible el código	<math>f(x) \,\!</math> <math>= \sum_{n=0}^\infty a_n x^n </math> <math>= a_0+a_1x+a_2x^2+\cdots</math>
Ecuaciones simultáneas	\begin{cases} 3x + 5y + z \\ 7x - 2y + 4z \\ -6x + 3y + 2z \end{cases}

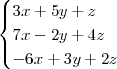Alfabetos

Alfabeto griego
\Delta \Theta \Lambda
\Xi \Pi \Sigma
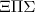\Upsilon \Phi \Psi \Omega
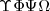\alpha \beta \gamma \delta \epsilon \zeta
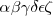\eta \theta \iota \kappa \lambda \mu
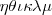\nu \xi \pi \rho \sigma \tau
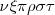\upsilon \phi \chi \psi \omega
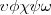\varepsilon \digamma \vartheta \varkappa
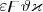\varpi \varrho \varsigma \varphi

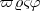Añadiendo paréntesis a grandes expresiones

	Sintaxis	Cómo se verá
Mal	( \frac{1}{2} )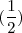
Bien	\left ( \frac{1}{2} \right )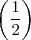

	Sintaxis	Cómo se verá
Paréntesis	\left ( \frac{a}{b} \right )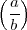
Corchetes	\left [ \frac{a}{b} \right ] \quad \left \lbrack \frac{a}{b} \right \rbrack
Llaves	\left \{ \frac{a}{b} \right \} \quad \left \lbrace \frac{a}{b} \right \rbrace
Barras y dobles barras	\left | \frac{a}{b} \right \vert \left \Vert \frac{c}{d} \right \|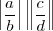
Barras invertidas	\left / \frac{a}{b} \right \backslash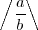
Flechas arriba y abajo	\left \uparrow \frac{a}{b} \right \downarrow \quad \left \Uparrow \frac{a}{b} \right \Downarrow \quad \left \updownarrow \frac{a}{b} \right \Updownarrow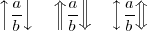
Los delimitadores pueden mezclarse	\left [ 0,1 \right )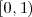
Usa \left. y \right. si no quieres que un delimitador aparezca	\left . \frac{A}{B} \right \} \to X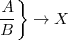
Tamaño de los delimitadores	\big( \Big( \bigg( \Bigg( ... \Bigg] \bigg] \Big] \big]
	\big\{ \Big\{ \bigg\{ \Bigg\{ ... \Bigg\rangle \bigg\rangle \Big\rangle \big\rangle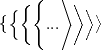
	\big\uparrow \Big\uparrow \bigg\uparrow \Bigg\uparrow ... \Bigg\Downarrow \bigg\Downarrow \Big\Downarrow \big\Downarrow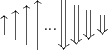
	\big\updownarrow \Big\updownarrow \bigg\updownarrow \Bigg\updownarrow ... \Bigg\Updownarrow \bigg\Updownarrow \Big\Updownarrow \big\Updownarrow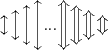
	\big / \Big / \bigg / \Bigg / ... \Bigg\backslash \bigg\backslash \Big\backslash \big\backslash

Espaciado

Nota: TeX elimina los espacios automáticamente, pero puedes controlarlos manualmente.

	Sintaxis	Cómo se verá
Espacio en blanco	a\ b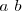

Ejemplos

Polinomio cuadrático	ax^2 + bx + c = 0\,\!
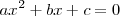Fórmula cuadrática	x=\frac{-b\pm\sqrt{b^2-4ac}}{2a}
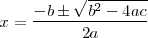Paréntesis altos y fracciones	2 = \left( \frac{\left(3-x\right) \times 2}{3-x} \right)
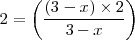	S_{new} = S_{old} + \frac{ \left( 5-T \right) ^2} {2}
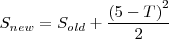Integrales	\int_a^x \int_a^s f(y)\,dy\,ds = \int_a^x f(y)(x-y)\,dy
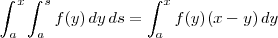Sumatorios	\sum_{m=1}^\infty\sum_{n=1}^\infty\frac{m^2\,n}{3^m\left(m\,3^n+n\,3^m\right)}
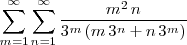Ecuaciones diferenciales	u + p(x)u' + q(x)u=f(x),\quad x>a
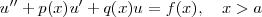Números complejos	|\bar{z}| = |z|, |(\bar{z})^n| = |z|^n, \arg(z^n) = n \arg(z)
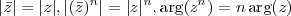Límites	\lim_{z\rightarrow z_0} f(z)=f(z_0)
Casos	f(x) = \begin{cases} 1 & -1 \le x < 0 \\ \frac{1}{2} & x = 0 \\ 1 - x^2 & 0 < x\le 1 \end{cases}

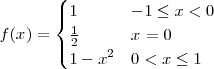Para más información visita la ayuda de TeX en Wikipedia