Teorema de Bayes
De Wikillerato
| Línea 59: | Línea 59: | ||
\cdot \mathrm{P} | \cdot \mathrm{P} | ||
\left( | \left( | ||
| - | \, B \, \left| \, A_1 \, \right | + | \, B \, \left| \, A_1 \, \right. |
\right) | \right) | ||
\, + \, | \, + \, | ||
| Línea 68: | Línea 68: | ||
\cdot \mathrm{P} | \cdot \mathrm{P} | ||
\left( | \left( | ||
| - | \, B \, \left| \, A_2 \, \right) | + | \, B \, \left| \, A_2 \, \right. |
| + | \right) | ||
\, + \, \ldots \, + \, | \, + \, \ldots \, + \, | ||
\mathrm{P} | \mathrm{P} | ||
| Línea 76: | Línea 77: | ||
\cdot \mathrm{P} | \cdot \mathrm{P} | ||
\left( | \left( | ||
| - | \, B \, \left| \, A_n \, \right | + | \, B \, \left| \, A_n \, \right. |
\right) | \right) | ||
} | } | ||
| Línea 188: | Línea 189: | ||
\left( | \left( | ||
\, B \, \left| \, A_2 \, \right. | \, B \, \left| \, A_2 \, \right. | ||
| + | \right) | ||
\, + \, \ldots \, + \, | \, + \, \ldots \, + \, | ||
\mathrm{P} | \mathrm{P} | ||
| Línea 210: | Línea 212: | ||
<br/> | <br/> | ||
| - | Tenemos tres | + | Tenemos tres urnas: |
<math> | <math> | ||
U_1 | U_1 | ||
</math> | </math> | ||
| - | con tres bolas rojas y | + | con tres bolas rojas y cinco negras, |
<math> | <math> | ||
U_2 | U_2 | ||
| Línea 292: | Línea 294: | ||
\right) | \right) | ||
} | } | ||
| - | \, = \, | + | \, = \, \frac |
| + | { | ||
| + | \frac{1}{3} \cdot \frac{3}{8} | ||
| + | } | ||
| + | { | ||
| + | \frac{1}{3} \cdot \frac{3}{8} \, + \, \frac{1}{3} \cdot \frac{2}{3} \, + \, | ||
| + | \frac{1}{3} \cdot \frac{2}{5} | ||
| + | } | ||
| + | |||
</math> | </math> | ||
</center> | </center> | ||
Revisión de 19:37 26 dic 2006
Tabla de contenidos |
Enunciado
Sean
 sucesos incompatibles dos a dos, tales que siempre ocurre alguno de ellos, y sea
sucesos incompatibles dos a dos, tales que siempre ocurre alguno de ellos, y sea
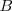 un suceso cualquiera del que se conocen las probabilidades condicionales
un suceso cualquiera del que se conocen las probabilidades condicionales
 .
.
Entonces las probabilidades
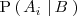 vienen dadas por la expresión:
vienen dadas por la expresión:

Demostración
Por definición de probabilidad condicionada
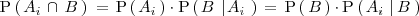
despejando
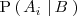 , se tiene:
, se tiene:
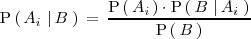
La probabilidad
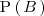 , por el teorema de la probabilidad total, es igual a
, por el teorema de la probabilidad total, es igual a
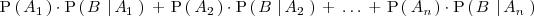
Sustituyendo en la ecuación anterior, obtenemos la fórmula de Bayes.
Ejemplo
Tenemos tres urnas:
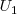 con tres bolas rojas y cinco negras,
con tres bolas rojas y cinco negras,
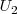 con dos bolas rojas y una negra y
con dos bolas rojas y una negra y
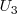 con dos bolas rojas y tres negras. Escogemos una urna al azar y extraemos una
bola. Si la bola ha sido roja, ¿cuál es la probabilidad de haber sido extraída de la urna
con dos bolas rojas y tres negras. Escogemos una urna al azar y extraemos una
bola. Si la bola ha sido roja, ¿cuál es la probabilidad de haber sido extraída de la urna
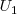 ?
?
Llamamos
 al suceso sacar bola roja. La probabilidad pedida es
al suceso sacar bola roja. La probabilidad pedida es
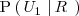 . Utilizando el teorema de Bayes, tenemos:
. Utilizando el teorema de Bayes, tenemos:
[Unparseable or potentially dangerous latex formula. Error 3 ]



